Give U A Banan

animation of the horses in love that I made

(i dont know how this social media works, SORRY 🐱 )

she delta on my epsilon til I converge

BUBSY?! LIKE FROM CARLETON?

:3 yes hiii

Here's another weird model of ZFC relating to the axiom of regularity that fucked me up when I learned about it. The axiom of regularity implies that there can be no set {x_n | n ∈ ω} such that x_{n+1} ∈ x_n for each n ∈ ω.

Let's construct our model. Add to the language of ZFC countably infinitely many constants c_0, c_1, c_2, ... and let Γ be the set of sentences Γ = {c_{n+1} ∈ c_n | n ∈ ω}. We will use the compactness theorem to show that there is a model of ZFC ∪ Γ.

Let Δ be a finite subset of Γ and Let J be any model of ZFC. Since Δ is finite, there is a maximum k such that the sentence c_k ∈ c_{k-1} is in Γ. Add to J the definitions, for each n ≤ k, c_nJ = k - n, and for each n > k, set c_nJ = 0. Then for all 1 < n ≤ k, c_n = k-n ∈ k-n+1 = c_{n-1}, and so J is a model of ZFC ∪ Δ.

Thus, by the compactness theorem, there exists a model of ZFC ∪ Γ.

This is very surprising, and at first glance seems to contradict the axiom of regularity! But what it really means is that the sets x_n from the first paragraph can exist, but they cannot be gathered together in a set.

downward lowenheim-skolem is so fucked up to me. what do you *mean* there's a countable model of first-order set theory

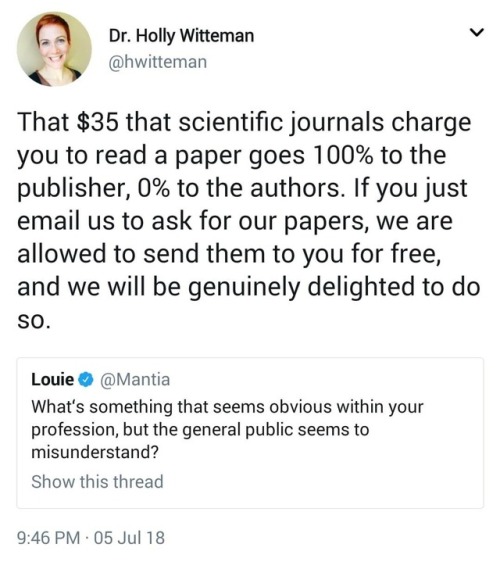
Found this on facebook but reposting to SAVE A LIFE.

Or at least some of y’all’s GPAs.

You’re welcome.

This is my new ciliate fursona :]

DERPY!!!!!!

tjhey are cousins in my au ok. thank you...

rainbow had blonde hair before the rainboom it was what dyed her mane.........and gave her her name... it was lightning dash before...

All mane 6 ships are created equal

Assorted pony doodles