Essa Animação é O Mesmo Modelo Descrito Na Publicação Abaixo 👇👇👇
Essa animação é o mesmo modelo descrito na publicação abaixo 👇👇👇
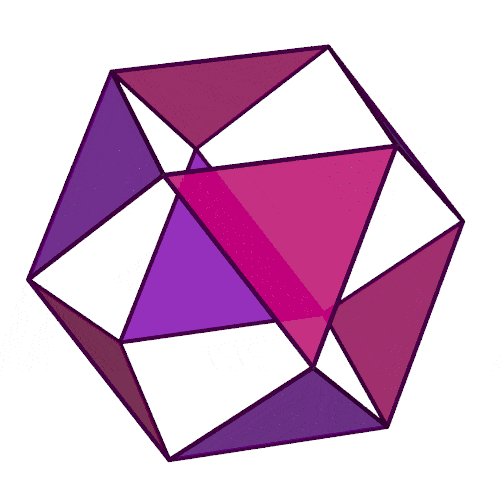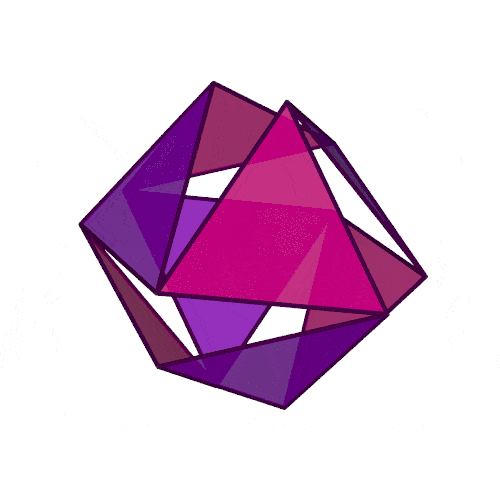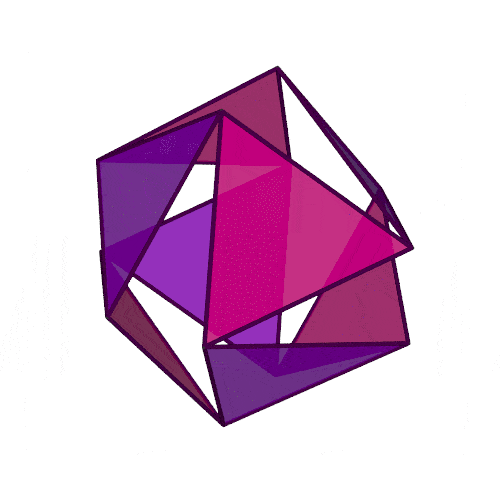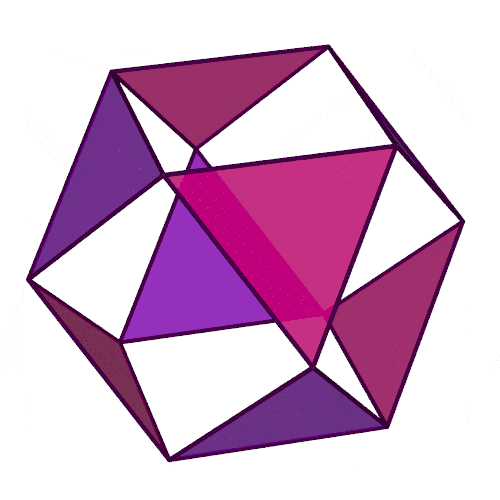

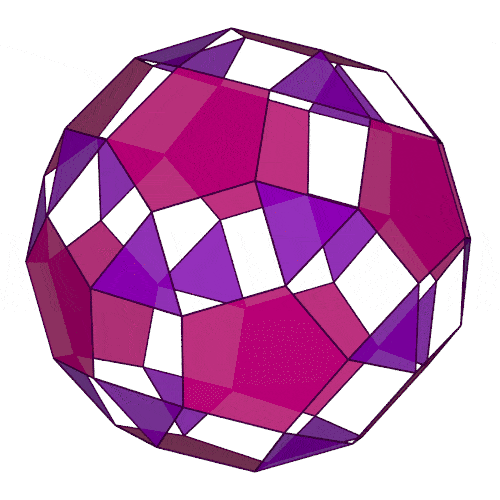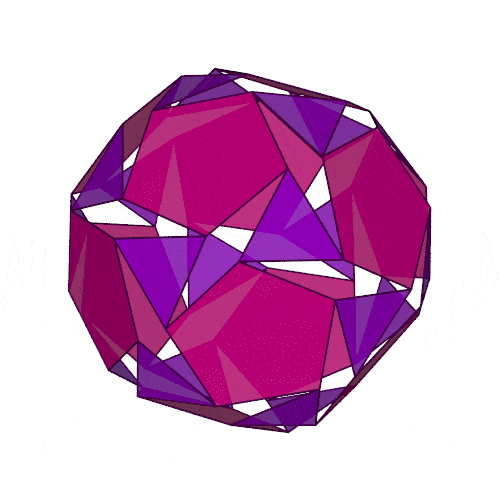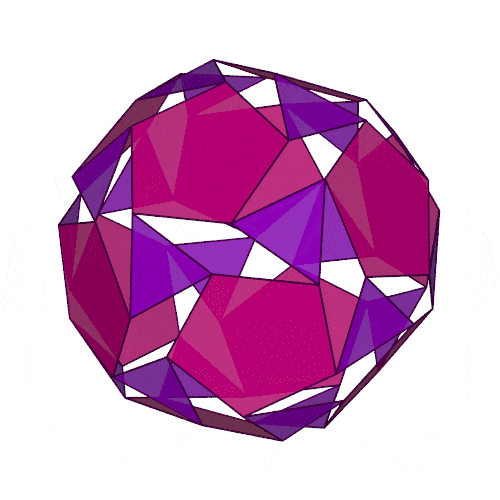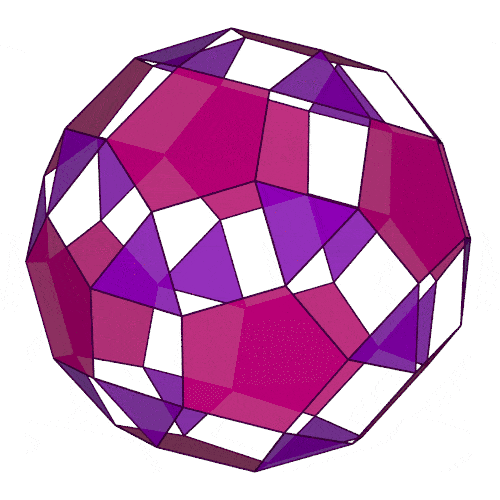
Semi-regular polyhedra transformations.
These transformations are same as the models in this post.
More Posts from Matematicaulysses and Others

INTERNATIONAL KWEEN OF TRIGONOMETRY




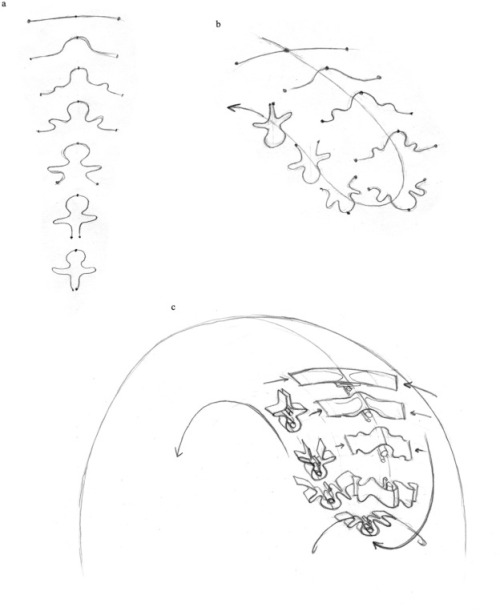





Embryo geometry: a theory of evolution from a single cell to the complex vertebrate body
24 “blueprints” that illustrate how the musculoskeletal, cardiovascular, neurological, and reproductive systems evolve through the mechanical deformation of geometric patterns. These images show how the vertebrate body might have evolved from a single cell during the evolutionary time and during individual development.
Though neither rigorous nor exhaustive in an empirical sense, our model offers an intuitive and plausible description of the emergence of form via simple geometrical and mechanical forces and constraints. The model provides a template, or roadmap, for further investigation, subject to confirmation (or refutation) by interested researchers.
The concept of “embryo geometry” suggests that the vertebrate embryo might be produced by mechanical deformation of the blastula, a ball of cells formed when a fertilized egg splits. As these cells multiply, the volume and surface area of the ball expand, changing its shape. According to the hypothesis, the blastula preserves the geometry of the initial eight cells generated by the egg’s first three divisions, which establish the three axes of the vertebrate body.
Though speculative, the model addresses the poignant absence in the literature of any plausible account of the origin of vertebrate morphology. A robust solution to the problem of morphogenesis—currently an elusive goal—will only emerge from consideration of both top-down (e.g., the mechanical constraints and geometric properties considered here) and bottom-up (e.g., molecular and mechano-chemical) influences.
Origin of the vertebrate body plan via mechanically biased conservation of regular geometrical patterns in the structure of the blastula, David B. Edelman, Mark McMenamin, Peter Sheesley, Stuart Pivar
Published: September 2016, Progress in Biophysics and Molecular Biology DOI: 10.1016/j.pbiomolbio.2016.06.007
A matemática é tão romântica!!!

Uma cidade perfeita para os exercícios de matemática!

Satellite map of Missoula, Montana
@vivalasestrellas




Toyota Matrix (matrix)

Agnes Denes. Isometric Systems in Isotropic Space: The Pyramid, 1976
Shearer’s geometry puzzles
On his blog MathWithBadDrawings, Ben Orlin reposted a couple of geometrical sangaku-like puzzles by math teacher Catriona Shearer. These are eleven of her personal favorites. If you dare, definitely give them a try!

Transit Across a Purple Sun. What’s the total shaded area?

Shearer’s Emerald. Four squares. What’s the shaded area?

The Pyramid with Two Tombs. Two squares inside an equilateral triangle. What’s the angle?

Setting Sun, Rising Moon. What fraction of the rectangle is shaded?

Hex Hex Six. Both hexagons are regular. How long is the pink line?

Four, Three, Two. What’s the area of this triangle?

The Trinity Quartet. All four triangles are equilateral. What fraction of the rectangle do they cover?

The Falling Domino. This design is made of three 2×1 rectangles. What fraction of it is shaded?

Slices in a Sector. The three colored sections here have the same area. What’s the total area of the square?
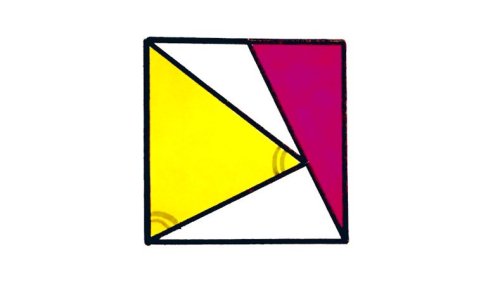
Disorientation. The right-angled triangle covers ¼ of the square. What fraction does the isosceles triangle cover?

Sunny Smile Up. What fraction of the circle is shaded?
More on the Spectre
Images from this great Yoshiaki Araki thread. (If you're interested in tessellations and are twitterpated, he's a must follow.)





He links the Nature article (good and a free read).
Best one, so far:

Robert Farthauer suggests:

And, of course, the actual article, by David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss.
Kaplan gives this illustration of the tile:

He has a one page stop for info about the tiling.
Bonus: via New-Cleckit Dominie

Calculating the surface area of a sphere. Found on Imgur.
-
 kkubaryi reblogged this · 3 weeks ago
kkubaryi reblogged this · 3 weeks ago -
 kkubaryi liked this · 3 weeks ago
kkubaryi liked this · 3 weeks ago -
 icalikesart reblogged this · 3 months ago
icalikesart reblogged this · 3 months ago -
 spacific-sunrise liked this · 3 months ago
spacific-sunrise liked this · 3 months ago -
 foundfootageromcom reblogged this · 3 months ago
foundfootageromcom reblogged this · 3 months ago -
 foundfootageromcom liked this · 3 months ago
foundfootageromcom liked this · 3 months ago -
 spookyinfluencertraveler liked this · 4 months ago
spookyinfluencertraveler liked this · 4 months ago -
 improbable-implosions reblogged this · 11 months ago
improbable-implosions reblogged this · 11 months ago -
 meadow-call reblogged this · 11 months ago
meadow-call reblogged this · 11 months ago -
 meadow-call liked this · 1 year ago
meadow-call liked this · 1 year ago -
 absolutesciencefiction reblogged this · 1 year ago
absolutesciencefiction reblogged this · 1 year ago -
 androidsghost liked this · 1 year ago
androidsghost liked this · 1 year ago -
 ceo-3dub liked this · 1 year ago
ceo-3dub liked this · 1 year ago -
 beachchairbookworm liked this · 2 years ago
beachchairbookworm liked this · 2 years ago -
 the-yearning-astronaut liked this · 2 years ago
the-yearning-astronaut liked this · 2 years ago -
 thoughtsformtheuniverse reblogged this · 2 years ago
thoughtsformtheuniverse reblogged this · 2 years ago -
 funkyfaerie liked this · 2 years ago
funkyfaerie liked this · 2 years ago -
 the-z-part reblogged this · 2 years ago
the-z-part reblogged this · 2 years ago -
 sezruedotter liked this · 2 years ago
sezruedotter liked this · 2 years ago -
 andrewndrag reblogged this · 2 years ago
andrewndrag reblogged this · 2 years ago -
 thoughtsformtheuniverse liked this · 2 years ago
thoughtsformtheuniverse liked this · 2 years ago -
 booksandchainmail reblogged this · 2 years ago
booksandchainmail reblogged this · 2 years ago -
 greeksandmagic liked this · 2 years ago
greeksandmagic liked this · 2 years ago -
 amygdalus liked this · 2 years ago
amygdalus liked this · 2 years ago -
 xaroof reblogged this · 2 years ago
xaroof reblogged this · 2 years ago -
 xaroof liked this · 2 years ago
xaroof liked this · 2 years ago -
 whiteground liked this · 3 years ago
whiteground liked this · 3 years ago -
 ettorestyle liked this · 3 years ago
ettorestyle liked this · 3 years ago -
 snapshot666-blog liked this · 3 years ago
snapshot666-blog liked this · 3 years ago -
 hebrideansky reblogged this · 3 years ago
hebrideansky reblogged this · 3 years ago -
 pictureimperfect liked this · 3 years ago
pictureimperfect liked this · 3 years ago -
 notclevr reblogged this · 3 years ago
notclevr reblogged this · 3 years ago -
 notclevr liked this · 3 years ago
notclevr liked this · 3 years ago -
 peachymean liked this · 4 years ago
peachymean liked this · 4 years ago -
 764647467 liked this · 4 years ago
764647467 liked this · 4 years ago -
 portablleek liked this · 4 years ago
portablleek liked this · 4 years ago -
 mountaingoat0112 liked this · 4 years ago
mountaingoat0112 liked this · 4 years ago -
 coffee-math-chamomile liked this · 4 years ago
coffee-math-chamomile liked this · 4 years ago -
 jeand54 liked this · 4 years ago
jeand54 liked this · 4 years ago
Blog do profº Ulysses TDBueno destinado a curiosidades, demonstrações, links, trabalhos, artigos, imagens e tudo que possa mostrar a matemática no mundo.
107 posts